Compound Planetary Gear
Planetary gear train with stepped planet gear set
Libraries:
Simscape /
Driveline /
Gears
Description
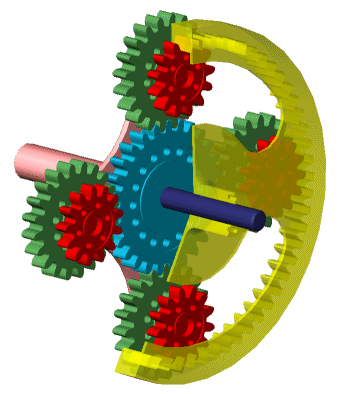
The Compound Planetary Gear block represents a planetary gear train with composite planet gears. Each composite planet gear is a pair of rigidly connected and longitudinally arranged gears of different radii. One of the two gears engages the centrally located sun gear while the other engages the outer ring gear.
Compound Planetary Gear
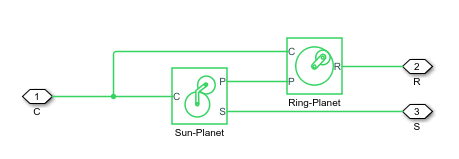
The block models the compound planetary gear as a structural component based on the Simscape™ Driveline™ Sun-Planet and Ring-Planet blocks. The figure demonstrates the equivalent block diagram for the Compound planetary gear block.
To increase the fidelity of the gear model, specify properties such as gear inertia, meshing losses, and viscous losses. By default, gear inertia and viscous losses are assumed to be negligible. The block enables you to specify the inertias of the internal planet gears. To model the inertias of the carrier, sun, and ring gears, connect Simscape Inertia blocks to ports C, S, and R.
Thermal Model
You can model
the effects of heat flow and temperature change by enabling the optional thermal port. To enable
the port, set Friction model to Temperature-dependent
efficiency.
Equations
The Compound Planetary Gear block imposes two kinematic and two geometric constraints.
where:
rC is the radius of the carrier gear.
ωC is the angular velocity of the carrier gear.
rS is the radius of the sun gear.
ωS is the angular velocity of the sun gear.
rP1 is the radius of planet gear 1.
ωP is the angular velocity of the planet gears.
rP2 is the radius of planet gear 2.
rR is the radius of the ring gear.
The ring-planet and planet-sun gear ratios are:
and
where:
gRP is the ring-planet gear ratio.
NR is the number of teeth on the ring gear.
NP2 is the number of teeth on planet gear 2.
gPS is the planet-sun gear ratio.
NP1 is the number of teeth on planet gear 1.
NS is the number of teeth on the sun gear.
In terms of the gear ratios, the key kinematic constraint is:
The four degrees of freedom reduce to two independent degrees of freedom. The gear pairs are (1, 2) = (P2, R) and (S, P1).
Warning
The gear ratio gRP must be strictly greater than one.
The torque transfers are:
and
where:
τP2 is torque transfer for planet gear 2.
τR is torque transfer for the ring gear.
τloss is torque transfer loss.
τS is torque transfer for the sun gear.
τP1 is torque transfer for planet gear 1.
In the ideal case where there is no torque loss, τloss = 0.
In the nonideal case, τloss ≠ 0. For more information, see Model Gears with Losses.
Assumptions and Limitations
Gear inertia is assumed to be negligible.
Gears are treated as rigid components.
Coulomb friction slows down simulation. For more information, see Adjust Model Fidelity.
Ports
Conserving
Parameters
More About
Extended Capabilities
Version History
Introduced in R2011a